Teacher package: Infinite series

The Plus teacher packages are designed to give teachers (and students) easy access to Plus content on a particular subject area. Most Plus articles go far beyond the explicit maths taught at school, while still being accessible to someone doing GCSE and A level maths. They put classroom maths in context by explaining the bigger picture — they explore applications in the real world, find maths in unusual places, and delve into mathematical history and philosophy. We therefore hope that our teacher packages provide an ideal resource for students working on projects and teachers wanting to offer their students a deeper insight into the world of maths.

Infinite series

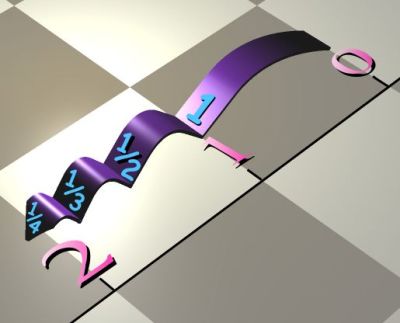
One of the first bits of school maths that gives us a real glimpse of infinity are infinite series: those never-ending sums that may nevertheless add up to a finite number. For this teacher package we've brought together all our articles on infinite series, grouped into the following categories:

• Is it finite?: Articles in this category give you explicit proofs of the convergence or divergence of some of our favourite series.
• Infinite series in mathematical action: These articles look at how series, their convergence or divergence, are used as tools in other mathematical results.
• Infinite series in real-life action: Here we explore how series can help us solve problems outside of maths.
• Infinite series and the biggest maths problem of them all: One famous series is the Riemann zeta function, which is involved in one of the biggest open problems in maths: the Riemann hypothesis. Articles in this category give you a glimpse of it.

Don't forget that our sister site NRICH has hands-on problems, activities and articles on series.

Is it finite?

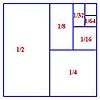
Outer space: Series — A visual and a mathematical proof of the convergence of our favourite geometric series.

In perfect harmony — Does the harmonic series diverge? Here's a proof.

An infinite series of surprises — This article shows us how eighteenth-century mathematician Leonhard Euler solved one of the foremost infinite series problems of his day.

Series in mathematical action

Mathematical mysteries: Zeno's paradox — This article shows how a geometric series helps solve one of the oldest paradoxes in maths, that of Achilles and the tortoise.

Beauty in mathematics — One of the most beautiful equations in maths, explored with infinite series.

Intriguing integrals — This article uses series to work out integrals in a clever way.

Making the grade — This article uses infinite series to create monstrous functions.

Series in real-life action

In perfect harmony — The harmonic series in action, from predicting rain fall to shuffling cards.

Have we caught your interest? — Using geometric series to work out compound interest.

Is this a record? — What the harmonic series can tell us about records.

No limits for Usain — Usain Bolt, the "fastest man on the planet", aims to get his 100 metre world record of 9.58 seconds down to 9.40 seconds. What has mathematics got to say about this quest? Another article involving the harmonic series.

Outer space: A collector's piece — How many cards do you need to buy to collect a set? Again, it's the harmonic series that has the answer.

Mathematical mysteries: The solitaire advance — Infinite series help with a particular challenge in the game of solitaire.

Infinite series and the biggest maths problem of them all

A whirlpool of numbers — An introduction to the Riemann hypothesis and the zeta function.

A disappearing number — A look at the maths behind the theatre play of the same name, which stunned the audience with the surprising result that 1+2+3+4+...=-1/12.

One L of a discovery — On the convergence and divergence of the Riemann zeta function and some of its cousins.