
Exotic spheres, or why 4-dimensional space is a crazy place
The world we live in is strictly 3-dimensional: up/down, left/right, and forwards/backwards, these are the only ways to move. For years, scientists and science fiction writers have contemplated the possibilities of higher dimensional spaces. What would a 4- or 5-dimensional universe look like? Or might it even be true, as some have suggested, that we already inhabit such a space, that our 3-dimensional home is no more than a slice through a higher dimensional realm, just as a slice through a 3-dimensional cube produces a 2-dimensional square?
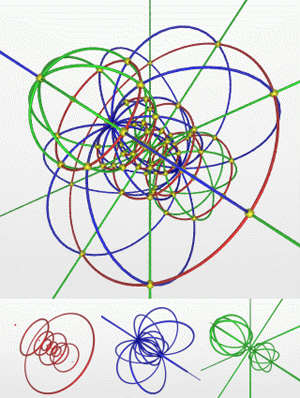
Just as a 3-dimensional object can be projected onto a 2-dimensional plane, so a 4-dimensional object can be projected onto 3-dimensional space. This image comes from the projection of a 4-dimensional hypersphere. The curves are the projections of the hypersphere's parallels (red), meridians (blue) and so-called hypermeridians (green). Image by Claudio Rocchini, via Wikimedia Commons.
According to the early 20th century horror writer H.P. Lovecraft, these higher dimensions do indeed exist, and are home to all manner of evil creatures. In Lovecraft's mythology, the most terrible of these beings goes by the name of Yog-Sothoth. Interestingly, on the rare occasions that Yog-Sothoth appears in the human realm, it takes the form of "a congeries of iridescent globes... stupendous in its malign suggestiveness".
Lovecraft had some interest in mathematics, and indeed used ideas such as hyperbolic geometry to lend extra strangeness to his stories (as Thomas Hull has discussed in Math Horizons). But he could not have known how fortunate was the decision to represent Yog-Sothoth in this manner. Strange spheres really are the keys to higher dimensional worlds, and our understanding of them has increased greatly in recent years. Over the last 50 years a subject called differential topology has grown up, and revealed just how alien these places are.
Higher dimensions and hyperspheres
Do higher dimensions exist? Mathematics provides a surprisingly emphatic answer to this question. Just as a 2-dimensional plane can be described by pairs of coordinates such as (5,6) with reference to a pair of axes, so 3-dimensional space can be described by triples of numbers such as (5,6,3). Of course we can continue this line of thought: 4-dimensional space, for a mathematician, is identified with the sets of quadruples of real numbers, such as (5,6,3,2). This procedure extends to all higher dimensions. Of course this does not answer the physicist's question, of whether such dimensions have any objective physical existence. But mathematically, at least, as long as you believe in numbers, you don't have much choice but to believe in 4-dimensional space too.
Well that is fine, but how can such spaces be imagined? What does the lair of Yog-Sothoth actually look like? This is a much harder question to answer, since our brains are not wired to see in more dimensions than three. But again, mathematical techniques can help, firstly by allowing us to generalise the phenomena that we do see in more familiar spaces.
An important example is the sphere. If you choose a spot on the ground, and then mark all the points which are exactly 1cm away from it, the shape that emerges is a circle, with radius 1cm. If you do the same thing, but in 3-dimensional space, we get an ordinary sphere or globe. Now comes the exciting part, because exactly the same trick works in four dimensions, and produces the first hypersphere.
What does this look like? Well, when we look at the circle from close up, each section looks like an ordinary 1-dimensional line (so the circle is also known as the 1-sphere). The difference between the circle and the line is that when viewed from afar, the whole thing curves back to connect to itself, and has only finite length. In the same way, each patch of the usual sphere (that is to say, the 2-sphere) looks like a patch of the 2-dimensional plane. Again, these patches are sewn together in a way that leaves no edges, and has only finite area. So far, so predictable, but exactly the same thing is true for the first hypersphere (or 3-sphere): each region looks just like familiar 3-dimensional space. We might be living in one now, for all we can see. But just like its lower dimensional cousins, the whole thing curves around on itself, in a way that flat 3-dimensional space does not, producing a shape with no sides, and only finite volume (you can find out more about the 3-sphere here). Of course we do not stop here: the next hypersphere (the 4-sphere), is such that every region looks like 4-dimensional space, and so on in every dimension.
From geometry to topology to differential topology
Like geometry, topology is a branch of mathematics which studies shapes. One of the fundamental questions to ask is when two shapes are really the same. This does not have a unique answer, it depends on the aspects of the shape that you are most interested in. At a basic level, if two shapes are identical, but are situated in different places, then for most purposes we will count them as being "the same".
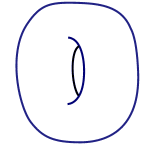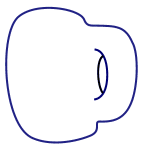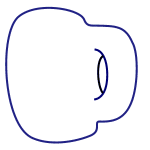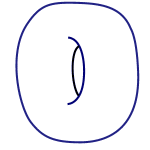
In topology a doughnut and a mug are the same because one can be morphed into the other.
Topology has a much broader notion of sameness than geometry. Here, two shapes are deemed "the same" if one can be pulled, stretched and twisted into the form of the other. So, to a topologist, triangles, trapeziums, septagons, and so on are all the same: they are all just circles. On the other hand, a figure of 8 is a genuinely different shape, because the topological definition of sameness never extends to cutting or gluing the shape. So an 8 can never be pulled into the shape of a circle as cutting is forbidden, and neither can a lower case i, as the two parts cannot be glued together.
If you are interested in things like angles, lengths, or areas, then the topological viewpoint is the wrong one. But a lot of important data is retained at this level: a famous example is the London tube map. Here, it is not the lengths or precise routes of the tunnels which matter, but things like the orders of the stations, and the ways that different tube lines intersect. These phenomena are topological in nature, and survive topological morphing. This is convenient, as it allows Londoners to use the famous simplified schematic map, rather than a detailed map of the whole city, incorporating the exact routes of all the tube lines.
Some shapes, such as the donut-shaped torus, have holes in them. These holes are essential; they cannot be removed by topological twisting or stretching. But which are the shapes with no holes? The most famous theorem in topology, the Poincaré conjecture, provides an elegant answer to this question: it says that the only such shapes are the spheres. This is not true from a geometrical viewpoint, as cubes, pyramids, dodecahedra, and a multidue of other shapes all have no holes. But, of course, to a topologist, all these exciting shapes are nothing more than spheres.
We have known since 2002 that the Poincaré conjecture is indeed true (find out more on Plus). Henri Poincaré's original question concerned the 3-sphere, but in fact exactly the same thing applies in all higher dimensions too. The fact is that, when viewed topologically, spheres are beautifully simple and unique objects in every dimension. However, in 1956 the first evidence arrived that a slight change in perspective would make the story hugely more complicated. When approached through the new subject of differential topology, higher dimensional spaces began to reveal some of their extraordinary secrets.
Gaps, kinks, and corners
The difference between plain topology and differential topology seems very subtle, but turns out to have astonishing consequences. It hinges on the precise type of pulling and stretching which are allowed during the morphing process. This has a dramatic impact on the shapes which are deemed to be "the same".

This is a fractal called a Julia set. Its outline is continuous, but nowhere smooth.
The divide is between processes which are continuous, meaning that they do not jump or tear, and others which are smooth. Smoothness is a much stronger condition than mere continuity. The same distinction applies to shapes themselves: circles and spheres are examples of smooth shapes, while squares and cubes are not smooth because of their sharp edges and corners. All of these are continuous, however, because their edges do not have any gaps or jumps. (A discontinuous line is one which comes in two separate pieces.) There are even fractal patterns which are continuous everywhere, but not smooth anywhere.
In the same way, we can distinguish between morphing which is truly smooth, and that which is merely continuous, but potentially very jerky and violent. It is not at all obvious, however, that this distinction should really matter very much. Might it really be possible that two shapes (also called manifolds by topologists) could be the same from a topological perspective (in technical terms, be homeomorphic), but not the same from a differential perspective (they are not diffeomorphic)? In other words, can we have two shapes that can be morphed into each other without cutting, but for which the morphing can't be smooth, it requires jerks and jumps? This is certainly difficult to imagine, not least because it never happens in dimensions 1, 2, or 3.
Exotic spheres
In 1956, John Milnor was investigating 7-dimensional manifolds when he found a shape which seemed very strange. On one hand, it contained no holes, and so it seemed to be a sphere. On the other hand, the way it was curved around was not like a sphere at all. Initially Milnor thought that he had found a counterexample to the 7-dimensional version of the Poincaré conjecture: a shape with no holes, which was not a sphere. But on closer inspection, his new shape could morph into a sphere (as Poincaré insists it must be able to do), but - remarkably - it could not do so smoothly. So, although it was topologically a sphere, in differential terms it was not.
Milnor had found the first exotic sphere, and he went on to find several more in other dimensions. In each case, the result was topologically spherical, but not differentially so. Another way to say the same thing is that the exotic spheres represent ways to impose unusual notions of distance and curvature on the ordinary sphere.
In dimensions one, two, and three, there are no exotic spheres, just the usual ones. This is because the topological and differential viewpoints do not diverge in these familiar spaces. Similarly in dimensions five and six there are only the ordinary spheres, but in dimension seven, suddenly there are 28. In higher dimensions the number flickers around between 1 and arbitrarily large numbers:
| Dimension | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 |
| Number of spheres | 1 | 1 | 1 | ? | 1 | 1 | 28 | 2 | 8 | 6 | 992 | 1 | 3 | 2 | 16256 | 2 | 16 | 16 |
The realm which remains the most mysterious, even today, is 4-dimensional space. No exotic spheres have yet been found here. At the same time no-one has managed to prove that none can exist. The assertion that there are no exotic spheres in four dimensions is known as the smooth Poincaré conjecture. In case anyone has got this far and is still not sure, let me make this clear: the smooth Poincaré conjecture is not the same thing as the Poincaré conjecture! Among other differences, the Poincaré conjecture has been proved, but the smooth Poincaré conjecture remains stubbornly open today.
The weird world of four dimensions

This is the projection involving a 4-dimensional object called a dodecaplex. Image crated by Paul Nylander.
So, is the smooth Poincaré conjecture true? Most mathematicians lean towards the view that it is probably false, and that 4-dimensional exotic spheres are likely to exist. The reason is that 4-dimensional space is already known to be a very weird place, where all sorts of surprising things happen. A prime example is the discovery in 1983 of a completely new type of shape in 4-dimensions, one which is completely unsmoothable.
As discussed above, a square is not a smooth shape because of its sharp corners. But it can be smoothed. That is to say, it is topologically identical to a shape which is smooth, namely the circle. In 1983, however, Simon Donaldson discovered a new class of 4-dimensional manifolds which are unsmoothable: they are so full of essential kinks and sharp edges that there is no way of ironing them all out.
Beyond this, it is not only spheres which come in exotic versions. It is now known that 4-dimensional space itself (or R4) comes in a variety of flavours. There is the usual flat space, but alongside it are the exotic R4s. Each of these is topologically identical to ordinary space, but not differentially so. Amazingly, as Clifford Taubes showed in 1987, there are actually infinitely many of these alternative realities. In this respect, the fourth dimension really is an infinitely stranger place than every other domain: for all other dimensions n, there is only ever one version of Rn. Perhaps after all, the fourth dimension is the right mathematical setting for the weird worlds of science fiction writers' imaginations.
About the author

Richard Elwes is a Visiting Fellow at the University of Leeds. He was the winner of the Plus New Writers Award 2006 and has since published articles in New Scientist and Daily Telegraph, and regularly talks about maths in public and on the radio. He is the author of the book Maths 1001, reviewed in Plus, and How to build a brain and 34 other really interesting uses of mathematics (March 2011)
Comments
Anonymous
You may now consider my mind bent to it's fullest! Thanks for fascinating intro into this very bizarre topological world!!
Anonymous
I saw a really beautiful and interesting documentary about this topic on:http://www.dimensions-math.org
Love the topic, made a movie about exploring the hidden dimensions in fractals myself:
http://www.vimeo.com/19251125
Wonder what you think of it.
Anonymous
Oh, this is just perfect! Who knew Lovecraft was a hard science fiction author? At any rate, I am now resolved to devote a bit more study to mathematics for my own writing as a result of this article, and I'm a guy who avoids math (history degree). Nicely done!
-Christopher Baughman
Anonymous
Thanks, Richard for putting all of this together for our entertainment. You keep the spirit alive. When the Old Ones return I am certain you will be compensated by being eaten first. Thanks again for upping the eldritch horror meter.
Anonymous
Great explanation. I went ahead and got your book. But I got lost right around where you explain what an exotic sphere is.
What do you mean my jerks and jumps? I asked my math major friend and couldn't quite understand the explanation. He said smooth means that "all of it's functions have derivatives in all orders." How does that relate to jumps and jerks?
What does "not diffeomorphic" mean?
Man I wish more Math was explained like this!! It sounds so interesting.
Anonymous
I have now a better understanding of math. Fractal thanks!
Anonymous
Nice article, but I'd like to see some exposition or some links to not-too-tech articles about the exotic spheres and the other types of 4d space. That's kind of the point of this article, I think, so you ought to show the readers where to get a real taste.
Anonymous
I am a student studying masters topology in my third year, and I stumbled across this article in hope of a clearer understanding to topological aims. I have to say the article is well written and has helped my perspective on higher dimensions. (I've always tried to imagine higher dimensions, but as you say our minds are only to think at most in the third.)
Thank you very much.
Anonymous
I am an eighth grade student and I agree, I am planning on becoming some sort of engineer and have always wondered about the dimensions higher than our own. Knowing that it is nearly, if not impossible to comprehend these planes feels pretty good.
Michael Reighn
The subject possible to explain from a mathematical standpoint, but is impossible to directly correlate it to anything tangible in this 3-dimensional world of ours. I would recommend trying to read these writings and writings of similar topics with a blank mind and try not to connect the ideas of 3d thought into something that directly defies it. That is what made me not understand the topic when I first started to dabble in it about a year ago. This can be thought about as both mathematically sound and abstract in the limits of our understanding. It’s one of the most confusing topics but also one of the most rewarding to understand!
Anonymous
taking 4-space in a piece wise fashion, it seems there are actually 6 (thats right; 6!!!) planes that are some how maybe mutually orthogonal to one another. Namely if we take four number lines and mark them w, x, y, z then we can construct the sub-space planes; (w,x), (w,y), (w,z), (x,y), (x,z), and (y,z). How can you have 6 mutually perpendicular planes in 4-space? Is it wrong to say that all the planes are mutually perpendicular? 4-space is turning out to be a really cool and interesting place indeed.
Anonymous
In 4-space there are only four perpendicular (or orthogonal) spaces, namely (w,x,y), (w,x,z), (w,y,z), and (x,y,z). Dimensionality is determined by the shape coordinates one dimension lower than the x-space that is being addressed. In 2-space and 3-space the shapes lower than x-space minus one don't result in enough perpendiculars to be addressed.
Anonymous
A single plane is defined by 2 perpendicular lines. Therefore, If you have n-dimensional space and thus n mutually perpendicular lines, you can make "n choose 2" (a binomial coefficient) different mutually perpendicular planes, which is equivalent to the (n-1)th triangular number, or to (n-1)n/2. It is for this reason that there is one plane in 2-d space, 3 planes in 3-d space, 6 in 4-d space, 10 in 5-d space, and so on. Might seem counterintuitive at first, but not after you invoke binomial coefficients!
Anonymous
The number of orthogonal k-dimensional spaces that "fit" in an n-space for any 0
Anonymous
Hi
Would 4 dimensional space as decribed here actually be 5 dimensions when you include time or is our world actually described by 4 dimensional space (including time)? Could this explain why time only ever moves in one direction (in contrast to the other dimensions)?
Thanks
Chavoux Luyt
Rrh
That's also what I'm wondering..if time is the 4th dimension then that leads me to believe that the 5th dimension is as different from the pervious four as time is from the pervious 3
Michael Reighn
To answer your question, time only moves in one direction from our point of view because we lack the ability to move through it freely. If you think about it from a 2 dimensional perspective, if a 3d sphere passed through the 2d plane, a 2d person would only see a circle growing bigger until stopping and start shrinking once again. This would be the cross-section of the sphere moving through it. The 2d plane would be “moving” through the height of the sphere just as we “move” through time/timespace. The 4th dimension is classified as “timespace” because it is a way to classify your position in not only physical 3 dimensional space, but time as well. I would definitely recommend looking up articles on something called “super-string theory.” It explains a theory that there is 10 dimensions, each with its own “pieces of the universal puzzle” if you will.
Anonymous
Thank you for the article. I'm wondering whether the geometry of a "sphere" in flat space 4D would include any corners? How about in the exotic R4s? If so, how many corners would there be?
Anonymous
I have tried to wrap my mind around differential topology several times over the past few years but never been able to do it well. And I am really confused now that I have read this article. My understanding was that DeRahm's theorem meant that any continuous morph on a smooth shape could be replaced by a smooth morph that does the same thing. But here we have spheres where that doesn't work.
Is there an intuitive explanation of how it really works (or a technical reference I can look up)?
Anonymous
Can't we just look at the 4th dimension from a 5th dimensional perspective, where everything is much simpler?
Just like you can study a circle and planar shapes from above in the 3d dimension?
If it's so difficult just give it an extra dimension for free.
Anonymous
A good aid in attempting to visualise higher dimensional form. In the table you list the numbers of types of spheres in various dimensions. I'm clear on 2D 3D having 1 type each, but have difficulty imagining a 1D sphere. Surely a singular dimension can contain no form and shouldnt be treated the same as combinations of dimensions, ie 2 and above, maybe I missed something. Fascinating and thought provoking, thank you
Anonymous
Think of a 3D sphere, slice it through the center. You now have a 2D "sphere" which actually looks nothing like a sphere, as your a whole dimension short. What im trying to prove here, is that you need to not get you definitions of words involved when thinking about this, think about it with an open mind. Now lets move to 1D. Essentially, turn a 2D "sphere" sideways. Now you have just a straight line. In a physical sence, a straight line is 2D. But think about it in the true sense, flat with no depth. Now this 3D sphere has entered the 1D space. Still a form of a sphere, just missing 2 dimensions. Now, is this 1D sphere any more obscure than the 2D? Not really. Its just that this image doesn't fit with your traditional thinking of a sphere and how it looks.
Josh Brown.
Queensland University of Technology
Davide Levi
A 1-D sphere is 2 points equidistant (by a radius) from a third point (the centre), not a line. Which is exactly what you get when you cut a circle with a plane passing through the centre of the circle, but not coplanar with the circumference. It is still the locus of all points equidistant from the centre.
Anonymous
A line is a filled-in 1D sphere, while two points is a hollow sphere. Generally, the word "sphere" refers to hollow spheres. A 3D filled-in sphere is known as a "ball," and a filled-in 2D sphere is called a "disc." I'm not sure what the general word for a filled-in sphere is.
Anonymous
So this might be simplistic but here it goes.
In2D or flatland, everything is only one point high, but different materials can exist. Such as Metal, concrete and black powder. If I make a Rectangular container put a tile in one half and black power in the remainder and lite it. Some of the explosion that doesn’t ‘disappear’ into the 3d space above would contact the tile. The tile would either 1) jiggle around and then come to a rest 2) be wedged upward and disappear into the 3d 3) have an edge remain in 2d and the rest be in 3d. It’s balanced on its edge.
Repeating this in 3d space with a wall in the shape of a rectangle, with a floor and roof this time so it’s enclosed. A cube is placed inside, and the remaining ½ filled with black powder. Results are 1) the cube Jiggles and comes to rest 2) the cube is reduced to bit’s.
The questions are
1) How much energy is needed to propel a 3d object into 4d space?
2) What material will survive the amount of energy directed at it?
3) Is this a plausible scenario?
Anonymous
I had a friend in high school that constructed for a Science Fair project what he called 'Visualizing A 4th. Dimensional Hypercube' using a record turntable and colored lights. It was a sight to behold the lights flashing on and off to represent the cube. Needless to say, everybody including the judges were awed. He was a genius. He taught me how to play chess. Another of his projects was a high voltage dielectric pump. He was bullied in school, and later in life tragically, committed suicide. RIP Jim.
Anonymous
I just wanted to say that I'm really sorry to hear that, not only because it is a tragic story, but because he sounds like the type of guy I would have got on with. I probably would have wanted putting in contact with him actually as I want to be able to visualise four-dimensional-constructions.
Again, my respect and condolences :(
Tom
Anonymous
It's a shame these types of things occur to brilliant people like your friend. Bully's are the ultimate degradation of progress in people's life. I am sorry for your lost friend.
Anonymous
Yog-Sothoth was a wimp. It's all about Azathoth, the Blind Idiot God. :)
Anonymous
It would be about Azathoth, if Azathoth was awake, but Azathoth being asleep is what allows existence to exist, hence why it has weird creatures playing it lullabyes and drum solos so it doesn't wake up and make everything go poof.
Anonymous
"But on closer inspection, his new shape could morph into a sphere (as Poincaré insists it must be able to do), but - remarkably - it could not do so smoothly. So, although it was topologically a sphere, in differential terms it was not."
Wait. I am certain that Poincaré himself never said a word about what is now called the "generalized Poincaré conjecture". His conjecture asked only whether a simply connected, compact 3-manifold is necessarily homeomorphic to the 3-sphere. (And in fact he expressed this solely as a question, without asserting that he believed it to be true.)
So, Poincaré never insisted anything at all about what a 4-connected 7-manifold might be able to do.
In fact, a little patient googling shows that the first statement of the generalized Poincaré conjecture (namely, that any compact n-manifold that is homotopy equivalent to an n-sphere is in fact homeomorphic to it) was given by the great topologist Witold Hurewicz in the mid-1930s.
Anonymous
He's obviously referring to the Poincaré conjecture and not Poincaré himself in that sentence.
Ali Lochhead
Thank you for such a clear and concise overview :-)
Four-dimensional
Rotation in four-dimensional space
https://youtu.be/R1rzI0Y_d3I
The 5-cell is an analog of the tetrahedron.
https://youtu.be/BjvdrhK8yws
Tesseract is a four-dimensional hypercube - an analog of a cube.
https://youtu.be/Pa0c7M4lZv0
The 16-cell is an analog of the octahedron.
https://youtu.be/np0ZxC1wXqc
The 24-cell is one of the regular polytope.
https://youtu.be/T01qw0_qitI
A hypersphere is a hypersurface in an n-dimensional Euclidean space formed by points equidistant from a given point, called the center of the sphere.
Anonymous
Thank you for reminding me about a course in topology I took over 60 years ago, involving shapes called POLYTOPS. It's pleasure reading articles so beautify written by this author.