This article is based on a London Mathematical Society popular lecture given by David Tong, Professor of Theoretical Physics at the university of Cambridge. You can see a video of the lecture below.
People often talk about the "unreasonable effectiveness of mathematics" — a phrase coined by the physicist Eugene Wigner in 1960 to capture the idea that mathematics describes the physical world far better than you'd expect from a mere human-made tool. Indeed, many physicists feel that mathematics expresses something deep about the nature of physical reality.
Why do they feel that? And can physics return the favour?
Unintended consequences
That mathematics should be useful in physics is no surprise. Whenever we need to measure, count, and understand patterns or relationships in the world, maths is an essential tool.
What is surprising, however, is that even mathematics that has been developed for the pure pleasure of pure maths can prove to be uncannily useful in physics, sometimes a long time after it was first thought of.
General relativity asserts that massive objects curve the fabric of spacetime. To formulate it Einstein used geometric notions of curvature developed by Riemann in the 19th century.
A fascinating example is a particular geometric notion of curvature developed by the mathematician Bernhard Riemann in the 19th century. Riemann cared nothing about physics when he came up with his ideas, and he certainly did not predict the dramatic developments in physics that were to flow from Albert Einstein's pen at the beginning of the 20th century.
Yet, Riemann's ideas turned out to be just what Einstein needed to formulate his general theory of relativity. According to general relativity, the force of gravity is the result of massive objects bending the fabric of spacetime. To describe this bending Einstein needed to define the curvature of a geometric object without reference to a surrounding space the object is embedded in — and this is exactly what Riemann had done before him. (See here to find out more about Riemann's notion of curvature.)
This is just one example of the unintended usefulness of mathematics. Purely mathematical considerations continue to lead the way in modern physics, and they continue to prove impressively productive (see here to find out more).
Simplicity
Once a mathematical description of a physical theory has been found, it is often surprisingly simple. This doesn't mean that the maths of physics is easy — far from it. It means that advances in physics don't come with ever more convoluted mathematics. Breakthroughs in physics happen when someone finds a new way of looking at a problem; a way that requires a mathematical framework that previously hadn't been considered for the purpose, or is completely new. Every single time such a new framework has been deployed in the history of physics, the simplest equation within it turned out to be the one that describes what is happening in our Universe.
Einstein's general theory of relativity is again a case in point. Its central equation is given below. Even if you don't understand what its symbols mean, you have to admit that for a description of all large-scale structures and processes in the Universe, it's elegantly brief. If you would like to understand the equation in more detail, see this article.
![\[ R_{\mu \nu } - \frac{1}{2}Rg_{\mu \nu } = \frac{8 \pi G_ N}{ c^4}T_{\mu \nu }. \]](/MI/cc5cd7e2a5f77f27248b5e7cd9237dbb/images/img-0001.png) |
Precision
Another thing that sets the mathematics of physics apart from maths as applied in other sciences is its incredible precision. As one example of many, consider a number called the 
factor, which serves to describe how the spin of an electron responds to an electromagnetic field. Experimentalists have measured the value of to be
![\[ g = 2.0023193043617 ± 3. \]](/MI/95edc6a0a3a3f766aa7d1f070dd6b0df/images/img-0002.png) |
It is also possible to calculate the value of using the mathematics that describes the electron and the force of electromagnetism. When you do this the value you get for is
![\[ g = 2.0023193043616… \]](/MI/95edc6a0a3a3f766aa7d1f070dd6b0df/images/img-0003.png) |
That’s an agreement to thirteen decimal places. In no other field of science do theory and experiment concord to such a spectacular extent.
Can physics give something back?
It can! Recent decades have seen an interesting new dynamic: ideas from physics have flowed across to mathematics and solved mathematical problems that had seemed completely inaccessible.
A beautiful example comes from physicists’ favourite approach to understanding unknown objects. They bombard an object with particles (which they do understand) and from the way these particles scatter off infer properties of the object. That's exactly what happens in particle colliders like the LHC.
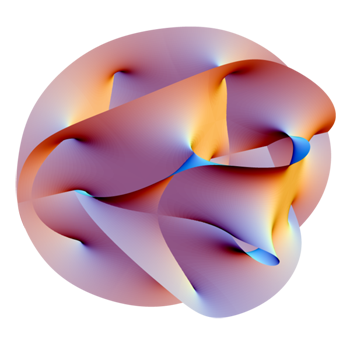
The Calabi-Yau manifold is one of the manifolds that benefited from the approach described here. To find out more about the Calabi-Yau manifold, see this article. Image: Lunch, CC BY-SA 2.5.
Since the time of Riemann mathematicians have been interested in manifolds. These are geometrical objects that, viewed from close up, look exactly like the ordinary Euclidean space we learn about at school. Their overall structure can be much more complicated than the plane or 3D space, however. They can even have more than three dimensions. And because we can't draw pictures of such manifolds or make them out of paper mâché, there are many things about them that mathematicians still don't understand.
This is where the scattering idea from physics comes in. Physicists allowed hypothetical particles, described by mathematical equations, to move around those abstract manifolds, allowing them to "feel" the space they were moving on. This proved particularly fruitful when they used hypothetical quantum particles, which can be in many places at once (see here), and the strings that replace the notion of particles in string theory. For example, strings enabled physicists to discover that certain manifolds come in pairs, a fact that had escaped mathematicians completely. The approach revolutionised geometry and answered questions in geometry that had been open for 100 years.
So do maths and physics really enjoy a special relationship? Is nature inherently mathematical? Or are these examples down to the way we choose to, or have evolved to, look at the world around us. These are questions for philosophers, which perhaps will find answers in the future.
To quote Eugene Wigner again:
"The miracle of the appropriateness of the language of mathematics for the formulation of the laws of physics is a wonderful gift which we neither understand nor deserve."
Comments
simplicity of mathematical language
I do not entirely disagree with the article but would like to point out that the apparent simplicity of mathematical formulae, like Einstein's field equations above, are to some extent illusory. Mathematics is a radical compression of logical and scientific language into a more economical set of conventional symbols, the meanings of which have evolved out of traditional use by specialized practitioners. Einstein is said to have called his summation notation his most significant contribution. I'm sure he said this tongue-in-cheek but it points to this important function of math practice. To the untrained, the field equations may appear simple, but they are completely mystifying because the layman does not possess the reams of background knowledge (which is not simple) to interpret the meaning of the symbols. These equations appear at once simple and meaningful only to the practiced expert who is able to implicitly expand their traditional symbols into the full and complex language of science and logic.
What if it's a dream
Given thhat we seem to live in a complex valued information space, where only observations are represented as real numbers, "particles" demonstrate unphysical behaviors, and even spacetime is being abstracted as ads-cft holographic math in order to explain away contradictions, given all of this...why should we continue to presume that there is a nature, or physical environment that we are witnessing. What if it's just one universal mental dream? Then it comes as no surprise that our most sophisticated mental processes become useful. When we naively believed in a local reality, our shared experience could be taken as evidence of a physical world, but that's no longer the simplest explanation. The simplest explanation is that while we are awake we are more fully integrated in a communal dream.
Backwards.
Suppose you collected for study that set of human experiences (i.e.: interactions with the world) for which mathematics proves particularly useful. And suppose you decided to call that “physics.” How far off would that be from what we actually do? I mean, when the math gets too messy or bogs down in ability to predict more, we set aside that set of experiences and call it chemistry or biology or psychology. In other words, there is no surprise finding math in physics if it essentially a defining characteristic of the field.
engineer
Knowledge as shown by science, is measurement. Measurement is a mapping of the world onto the number line - a sequence of numbers. Our brains have evolved to make sense of scattering events of photons - arranging these events into sequences, so as to plan actions that help in survival and reproduction. Sequences therefore is knowledge. To arrange these huge numbers of sequences and make predictions, our brains have evolved concepts like space and time. Space and time are not physical entities, but useful concepts. When physicists make a space-time description of the universe, they forget that space and time are merely concepts. If you get rid of the notion of space and time and merely look at physics as a description of sequences of scattering events that leads to predictability - a non-spacetime physics, then the connection between mathematics i.e., logical conclusions of a prototype sequence, and physics becomes apparent. And perhaps lead to describing physical laws in simpler mathematics.