Article


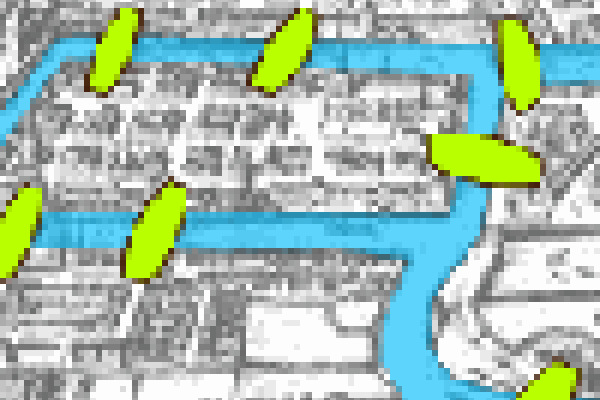
The bridges of Königsberg
Can you find a path through on this city map that crosses every bridge exactly once? Euler's answer to this problem started off the filed of graph theory.